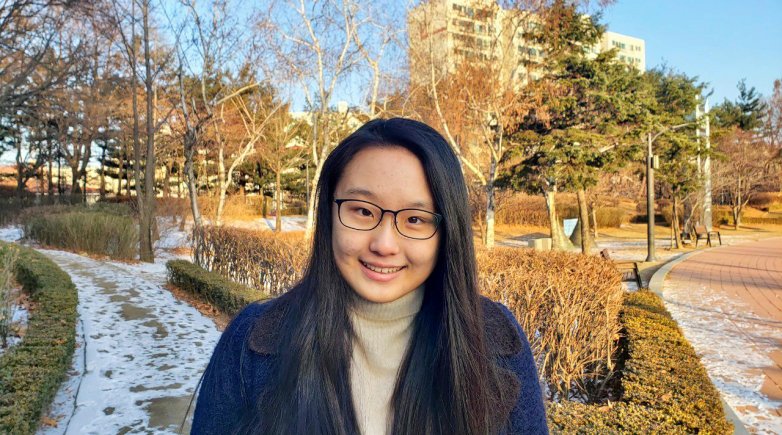
Exeter senior awarded nation's top science prize
Yunseo Choi '21 claims $250,000 prize as winner of 80th Regeneron Science Talent Search.
Yunseo Choi '21 joined the ranks of 13 Nobel Prize winners and scores of the nation's top science minds Wednesday night, taking the top spot in the 80th Regeneron Science Talent Search.
Choi, a resident of Seoul, Korea, and four-year Exeter student, outlasted 39 finalists from across the United States to claim a $250,000 winner's prize. The award ceremony was held remotely and streamed live.
Choi's project “On Two-Sided Matching in Infinite Markets” had been chosen in January as a finalist in the STS, the nation’s oldest science and mathematics competition for high school seniors. Finalists were selected from more than 1,700 entrants.
Each finalist already had secured at least $25,000, with the top 10 prizes ranging from $40,000 to $250,000. The top 10 winners were identified — counting down from 10 — at the conclusion of the hour-long ceremony Wednesday night.
For her #RegeneronSTS project, @PhillipsExeter student Yunseo Choi researched matching theory. The project helped her win first place and $250,000. https://t.co/dBfmJBFYkh pic.twitter.com/Q5n9AgRdOK
— Society for Science (@Society4Science) March 18, 2021
Choi’s project aims to build off the Gale-Shapley algorithm which seeks to solve the problem of finding a stable match between two equally sized sets of elements. The two California professors for whom the algorithm is named proved in 1962 that, for any equal number of men and women, it is always possible to find matches to make all marriages stable.
“Gale and Shapley proposed a seemingly impossible question: ‘How can we form stable marriages?’ (as if they had watched any season of The Bachelor!),” Choi wrote in an email in January after she was selected as a finalist. “Now, of course, this question cannot be answered with mathematics alone. However, matching theory begins to answer this question by characterizing the problem through models of men and women and their preferences on each other.”
Economic theorists have since applied matching algorithms in countless ways.
“Matching markets dominate the world, from Tinder to Airbnb,” Choi said. “Economic theory seeks to understand the properties of these markets, but some theory predictions are wrong because they depend on unrealistic assumptions. How do we find the most fundamental results? Go infinite!
“My project seeks to characterize the properties of matching that are the most fundamental. By characterizing these results, I aim to help design matching algorithms that are more robust to frictions in the market that are not fully captured by the restrictive finite models. This created the perfect opportunity to translate the beautiful mathematics in these models into a result that is important for practical market design.”
Selection as a finalist triggered an arduous round of judging from a panel of 16 scientists. Each finalist was required to present their project to the panel and was subject to a rigorous round of interviews by the judges.
Choi, who hopes to be a theoretical economist some day, credits her teachers and mentors — including Exeter Math Instructor Jeff Ibbotson and a cast of college mathematicians, including Scott Duke Kominers at Harvard, where she’s headed in the fall.
“Without them, none of this would have been possible,” she said. “Although progress should never be judged by the resulting outcome alone, being named a Regeneron STS Finalist is such an incredible honor.”
“Yunseo is a fabulous student and always approaches mathematics with a depth of insight and continuing interest in various subfields of math,” Ibbotson said. “She loves sharing new proofs of well-known results and she is constantly thinking about extensions of the things she has learned. She truly lives the life of a mathematician!”